
Learning Resources
Lesson

Again, this Investigation is self explanatory. You are given a description of the price of tickets for a whale-watching tour. Pay particular attention to the special rate offered to groups of more than 30. Your task will be to determine the number of passengers that will give the greatest revenue. Be sure to keep in mind that:
Total Revenue = # of passengers TIMES the cost per ticket.
Begin the Investigation as outlined on pages 60 and 61 of your text. If graphing technology is used, the graph on the screen must be copied into your notebook. You should progress through steps A to F easily. However, hints are provided for Steps F and G, should you experience difficulty. Keep in mind, as well, that throughout the entire procedure, x represents the number of passengers in excess of 30.
The graph of the product of two functions can be found on a graphing calculator. Click here if you would like a detailed explanation of this procedure.
Now you are ready to do the Investigation Questions on page 61. Complete numbers 15 to 18.
Activity
C.Y.U. pages 19 - 23 #'s 19 -23
When you have completed these questions, ask your on-site teacher to get the solutions for you from the Teacher's Resource Binder and check them against your answers. After you do this, if there is something you had trouble with and still do not understand, contact your on-line teacher for help.
Test Yourself
- Given f(x) = x + 3 and g(x) = 2x - 4. Predict the shape of the graph of f(x) x g(x). Also state its domain, range and intercepts.
- For each of the following, state non-constant functions from which each could have been formed, using combination by multiplication.
(a) f(x) = x2 - 8x + 15 (b) g(x) = 3x2+ 18x - 21
Solutions
1. The graph will be a parabola, since a quadratic is the product of two linear
equations.
Domain: {x / x ? R}
To find the range, you need the vertex of the parabola. 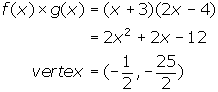
Thus, the range is ![]()
y-intercept: -12
x-intercepts: 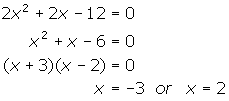
2. (a) x2 - 8x + 15 = (x - 5)(x - 3) Thus, f(x) was formed from functions g(x) = x - 5 and h(x) = x - 3.
(b) 3x2+ 18x - 21 = (3x - 3)(x + 7)
Thus, g(x) was formed from the functions f(x) = 3x - 3 and h(x) = x + 7.
Notice that the common factor of 3 was not removed before the
expression was factored. If so, there would have been a constant
function of 3 involved. The question indicated that all functions were to be
non-constant.