
Learning Resources
Outcomes
In this lesson you will learn
- how pressure and volume of a gas are related
- the terms horizontal asymptote and vertical asymptote
- the behaviour of functions of the form
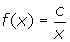
- the difference between an odd and even function
By the end of this section students will be able to:
- model real-world phenomena using polynomial functions and rational functions
- model problem situations with combinations and compositions of functions
- analyze relations, functions, and their graphs
- use tables and graphs as tools to interpret expressions
- investigate and interpret combinations and compositions of functions
- demonstrate an understanding for asymptotic behaviour
- analyze and solve polynomial, rational, irrational, absolute value, and trigonometric equations
Introduction
This section is intended to allow you to examine the behaviour of a simple rational function. You will explore the functions ![]() and
and ![]() .
.
Once you have established patterns, you will use this knowledge to assist you in using the graph of a function such as f(x) = x + 1 to graph its reciprocal ![]() .
.
It follows then that you should be able to use the graph of f(x) = x2 + 1, for example, to graph the reciprocal function ![]() .
.
The understanding of this concept will prepare you to deal with more general rational functions in which the numerator is not a constant, to be introduced in the next section.
This section of study requires about 4 hours for completion.
Prerequisites
To be successful in this lesson, it would be helpful to know the following:
- the concept of reciprocal
- domain and range
- limits
- transformations
- how to graph a scatter plot
- how to do a regression analysis on the graphing calculator
- how to write the equation of a vertical line