Learning Resources

a) will have two equal real roots when . From the equation we note that a = 1,  b = k and c = - k + 8. Substitute and solve for k.

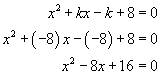
For k = -8 and k = 4 the equation will have two real equal roots. These equations can be found by substituting the values into the equation.

For k = -8 

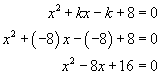
For k = 4

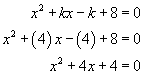
b) For two unequal real roots, the discriminant must be greater than zero. Using the results from (a) any value of k such that k > 4 or k < - 8 will ensure that the discriminant is positive.

c) For two imaginary roots, the discriminant must be less than zero. Any value k such that - 8 < k < 4 will ensure that the discriminant is negative.