Learning Resources

Lesson

First we want to consider an angle as having two arms which meet at a fixed point called the vertex. We will consider one of the arms to be fixed in place and the other free to rotate. The fixed arm is called the initial arm or initial side and the rotating arm is called the terminal arm or terminal side. Click here to see the diagram. Click on the point on the terminal side to rotate it.

If we now put this angle on a coordinate system with its vertex at the origin and the initial arm along the positive x-axis, we say that the angle is in standard position. This is shown in the diagram below.

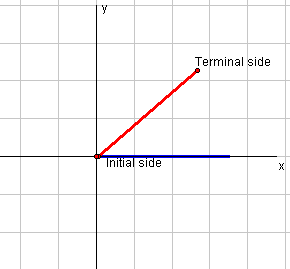
Standard position

We are interested in the coordinates of the point where the terminal arm of the angle in standard position intersects a circle whose centre is at the origin of the same coordinate system. This is shown in the diagram below.

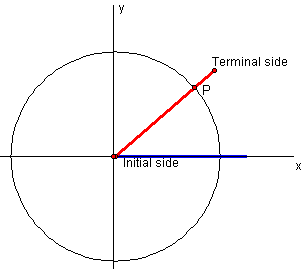
We want to determine the coordinates of P. The method of determining these coordinates is shown below.

To determine the coordinates of point P, construct a perpendicular to the x-axis from P and consider the right triangle so formed. This is shown in the diagram below: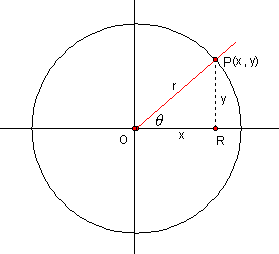

For convenience purposes, small letters are used to denote the sides of the angle and q is used to denote the central angle.

Consider DPOR. From our previous work on trigonometry we know the following:

By multiplying both sides by r we get:

Thus the coordinates of the point where the terminal arm intersects the circle can be given as (r cos q, r sin q). Two things thus affect the coordinates of the point. They are the length of the radius and the measure of the angle. This is illustrated by the following example.

Example

Find the coordinates of the point where the terminal arm intersects the circle for the following conditions:

1. Radius = 4, central angle = 35°
2. Radius = 7, central angle = 73°

Solution

1. x-coordinate = r cos q = 4 cos 35° = 4 (0.8192) = 3.276
   y-coordinate = r sin q = 4 sin 35° = 4 (0.5736) = 2.294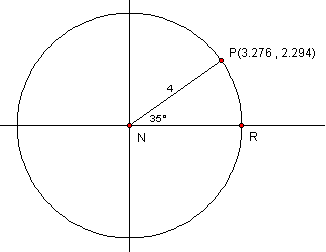
   
   Note: When using your calculator to find the sine or cosine of an angle, make sure it is set to degrees and not radians. You determine this by pushing the [mode] button and highlighting degrees.
   
2. x-coordinate = r cos q = 7 cos 73° = 7 (0.2924) = 2.047
   y-coordinate = r sin q = 7 sin 73° = 7 (0.9563) = 6.694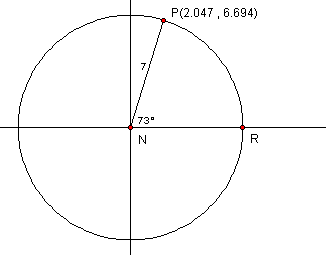
   

So far we have only considered an acute angle which has its terminal arm in the first quadrant. We now want to turn our attention to angles whose terminal sides are in the other quadrants.

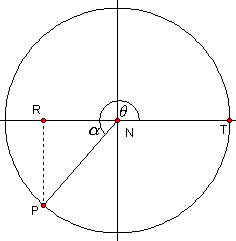
When we construct the perpendicular to the x-axis from a point where an angle larger than 90° intersects the circle, the triangle formed does not contain the required angle. This is illustrated in the diagrams below. In all of the . examples, q , the angle in standard position in which we are interested, is not contained in the triangle formed. However, a related angle, a is formed. It is the angle between the terminal arm and the x-axis.

related angle a = 180° - q

related angle a = q - 180°

related angle a = 360° - q

Example

For each case, construct a diagram and indicate on it the angle in standard position and the perpendicular to the x-axis. Then find the related angle and use it to find the coordinates of the point where the terminal arm intersects the circle:

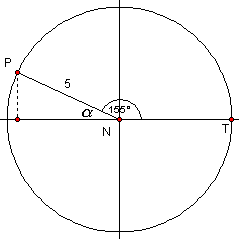
1. Radius = 5, central angle = 155°
2. Radius = 9, central angle = 228°
3. Radius = 3, central angle = 326°

Solution:	Related angle a = 180°-155° = 25° r cos a = 5 cos 25° = 5 (0.9063) = 4.53 r sin a = 5 sin 25° = 5 (0.0.4226) = 2.11 Since the point is in the second quadrant, the x-coordinate must be negative and y-coordinate positive: P has coordinates (-4.53 , 2.11)
2.	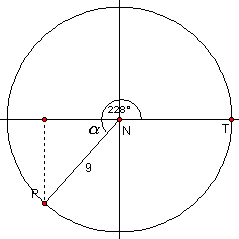Related angle a = 228°-180° = 48° r cos a = 9 cos 48° = 9 (0.6991) = 6.02 r sin a = 9 sin 48° = 9 (0.7431) = 6.69 Since the point is in the third uadrant, the x-coordinate must be negative and the y-coordinate must be negative: P has coordinates (-6.02 , -6.69)
3.	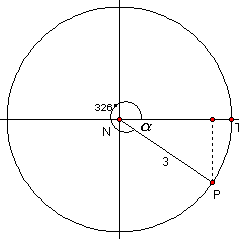Related angle a = 360°-326° = 34° r cos a = 3 cos 34° = 3 (0.8290) = 2.49 r sin a = 3 sin 34° = 3 (0..5592) = 1.68 Since the point is in the fourth quadrant, the x-coordinate must be positive and the y-coordinate must be negative: P has coordinates (2.49 , -1.68)

Before you go to the activities for this lesson, redo the above examples by substituting the given angles directly in the formulas and calculating the coordinates of the points using them instead of first finding the related angle. For example, on your calculator, enter 155° , 228° , 326°. What did you notice? Why then did we bother with related angles? The answer to that will be seen in the next lesson.

Activity

1. Do the Investigation Questions 3 - 5 on pages 270 & 271 in your text

When you have completed these questions, ask your on-site teacher to get the solutions for you from the Teacher's Resource Binder and check them against your answers. After you do this, if there is something you had trouble with and still do not understand, contact your on-line teacher for help.

Test Yourself

An angle of 118° is drawn in standard position. Two circles with centres at the origin are drawn, one with a radius of 4 and another with a radius of 8. Find the coordinates of the points where the terminal arm of the angle intersects the circles.

Solution