Learning Resources

Lesson

In previous courses you studied the equations of lines and parabolas. There you learned that there is a basic form for the equation of each of these figures. For example you learned that one form for the equation of a line is y = mx + b, and one form for the equation of a parabola is y = a(x - h)²+k.

We now wish to establish the form of an equation for a circle. We will start with the simple case of a circle with centre at the origin. Later, we will transform this graph and see what happens to the equation. However, for now, let's work with a circle with centre at the origin.

What we are looking for is an equation that gives the relationship between the x and y coordinate of any point on a circle. In the diagram below, this means how x is related to y:

This is what is developed in Investigation on page 252 in your text. Turn to it now and complete the steps as directed. Hints are provided for some of the steps that may prove difficult.

• For hints and comments on Step B click here.
• For hints and comments on Step C click here.

Do not continue past this point until after you have completed Steps A to H of Investigation 6 on page 252 in your text.

In the investigation you should have determined that the form for the equation of a circle with centre at the origin and radius r is:

x² + y² = r²

To investigate as many examples of this equation as you wish, view the following interaction using Geometers Sketch Pad. Remember, once you have started to do the investigation, the diagram may get messed up. If it does, to return to the original screen you started with, simply press r. This will restore the screen to the original diagram. When you are finished with the investigation, simply click the [back] button to returned to this page.

To investigate as many examples of this equation as you wish, view the following interaction using Geometer?s Sketch by clicking here.

To determine if a given point is inside, on, or outside a circle, we have to determine its distance from the centre of the circle and compare it to the radius of the circle.

Example

Where is the point A(5 , 3) in relation to the circle with equation x² + y² = 36?

Solution

The circle with equation x² + y² = 36 has centre at the origin and radius 6. The distance from A to the centre (0 , 0) is: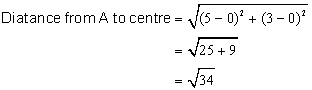

Since , the point is inside the circle.

We now want to consider the equations we have been studying as transformations of the unit circle which has equation x² + y² = 1. What we are doing is stretching this basic graph both vertically and horizontally by a factor which corresponds to the radius of the circle. If we only stretch in one direction, either vertically or horizontally but not both, the figure is no longer a circle. We will speak more of this in a later lesson.

Example

Describe x² + y² = 144 in terms of a transformation of the unit circle and write the mapping rule for the transformation:

Solution

Since the equation is x² + y² = 144, the radius is 12 and the unit circle is stretched both horizontally and vertically by a factor of 12. We can write the mapping rule as follows: .

You should now be ready to do the work associated with this lesson.

Activity

1. If you have not already done so, complete Investigation 6 on page 252 in your text.
2. Complete Investigation Questions 1 & 2 on page 253.
3. Do the CYU Questions 3 - 6 on page 253.

When you have completed these questions, ask your on-site teacher to get the solutions for you from the Teacher's Resource Binder and check them against your answers. After you do this, if there is something you had trouble with and still do not understand, contact your on-line teacher for help.

Test Yourself

The equation of a circle is x² + y² = 65

1. What are the coordinates of its centre and the length of its radius?
2. Sketch the circle on grid paper.
3. Where is the point (5 , 6) in relation to the circle?
4. Describe the circle in terms of a transformation of the unit circle and write the mapping notation for it.

Solution