Learning Resources
Lesson
In this lesson you will do proofs that require the properties of tangents. These proofs will also require many of the other properties about chords, inscribed angles, congruent triangles, etc. that we have discussed so far.
After you are familiar with the various properties, the best way of learning to do proofs is to observe some examples of them and then to practice doing them yourself. With this in mind, work through the following example. Before you go on to each step in the example, decide what you would have written and compare it to what is presented.
Example
Prove that the segment joining the point of intersection of two common tangents to the centre of a circle bisects the angle between the tangents.
Given: BC and BD are tangents
Prove: Ð ABC @ Ð ABD

To see the proof developed in a step by step fashion click here.
Another example of proof with tangents is provided in Example 9 in your text on page 245 (remember we do not do the transformational method of the proofs).
Activity
- Study Example 9 on page 245 in your text.
- Do CYU Questions 52 - 54 & 56 - 58 on pages 246 - 247.
When you have completed these questions, ask your on-site teacher to get the solutions for you from the Teacher's Resource Binder and check them against your answers. After you do this, if there is something you had trouble with and still do not understand, contact your on-line teacher for help.
Test Yourself
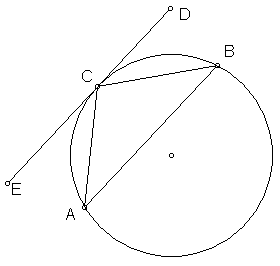
In the diagram below, it is given that ED is a tangent to the circle at point C and AC @ BC. Prove that ED || AB