Learning Resources

Lesson

In this lesson, instead of doing an investigation and making a conjecture about the properties of arcs, chords, inscribed angles, etc., we will consider some of these same properties (and many new ones) and provide a Euclidean proof for them.

The only way to become proficient with proofs is to practice writing them and study examples of how they are set up. However, if you do not memorize the properties as you learn them, you will not be able to apply them in future proofs. Before you begin, you might like to quickly review what you have done so far in this Unit.

Example 1

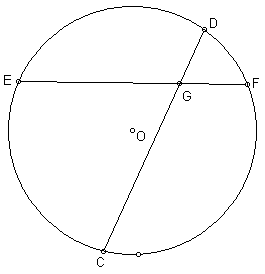
The first example we will do is Focus Question 18 (d) on page 238 in your text. It asks us to prove that an angle formed by two chords intersecting inside a circle is half the sum of the arcs intercepted by the angle and its vertically opposite angle. This is shown in the diagram below

Given:

Chords EF and CD intersecting at point G.

Prove:

m ÐCGE = ½ (m + m )

Proof:

To see the proof presented in a step by step fashion click here.

Note that in the above diagram we can also say that:

m ÐDGE = ½ (m + m )

In addition to the proofs associated with this lesson, there are several problems which require calculations. Some of these calculations require formulas that you learned in previous courses. A few of the formulas are given in the margins in your text book, the others you will have to recall or look up again. The next page deals with one problem of this type.

Example 2

A chord 4.8 cm long is drawn in a circle of radius 3.0 cm. What is the area of the minor segment cut off by this chord?

Click here to see the solution.

There were a lot of new terms in this lesson. The definitions for all of them are in the margins in your text book. Be sure you know the meaning of the following terms: cyclic quadrilateral, tangent, secant, sector of a circle, and segment of a circle.

You should also be able to do Euclidean proofs and calculations using the properties of chords and arcs.

Summary

1. You should know the meaning of the following terms: cyclic quadrilateral, tangent, secant, sector of a circle, segment of a circle. Check the definition for these terms in your book.
2. You should be able to do Euclidean proofs and calculations using the properties of chords and arcs.

Activity

1. Complete Focus E on pages 237 & 238 in your text.
2. Do the Focus Questions 18 (a) - (e), 19 - 23 on pages 238 & 239.
3. Do the CYU Questions 24 - 32, 34 - 37, 40 - 46 on pages 240 - 243.

When you have completed these questions, ask your on-site teacher to get the solutions for you from the Teacher's Resource Binder and check them against your answers. After you do this, if there is something you had trouble with and still do not understand, contact your on-line teacher for help.

Test Yourself

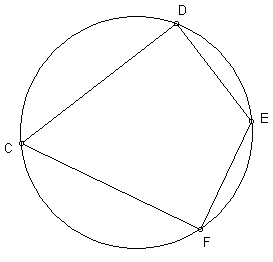
1. In the diagram below, DE @ FE and DC @ FC. Prove: @

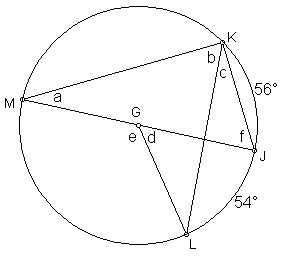
2. In the diagram below, m = 56° and m = 54° Find: a, b, c, d, e, f

Solution