Learning Resources

Lesson

If you know the vertex and at least one other point of a parabola you can find its equation using the transformational form of the quadratic function. You may get the vertex and the point directly from the graph or you may get the information from some other source.

Regardless of how you determine the vertex and the other point, once you have them there are two ways to proceed to find the equation. These two methods are shown by example in Focus E on pages 36 and 37 in your text book. Pick the method which you find the easiest and use it to answer the assigned questions. Another example is done for you below. Study the two methods as you should be able to follow both.

Example

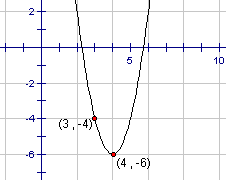
Find the equation of a parabola with vertex (4, -6) and passing through the point (3, -4).

Solution

You may want to draw a sketch of the parabola before you start. It helps to see what is happening:

Method 1 : Start with the equation

The vertex is (4, -6). This represents a horizontal translation of 4 units right and a vertical translation of 6 units down. These translations applied to give the following equation:

Now compare the patterns for the new function with . Since the second point, (3 , -4), is 1 unit to the left of the vertex and 2 units above it , we have the following pattern:

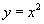• over 1 up 1

• New function over 1 up 2

Therefore, the vertical stretch is 2 upward which means a is positive.

The quadratic function describing the parabola will be

Method 2 : We know the transformational form of a quadratic equation is where (p, q) represents the vertex of the parabola. From the problem we have the vertex (4, -6) and substituting this into the equation we get:

To find the value of k, substitute the values for x and y from the second point (3, -4) into the equation. This gives:

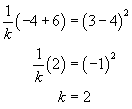
Substituting into the transformational form we have

As was stated above, use whichever method you are most comfortable with to answer the questions in the text. However, Method 2 may prove a little easier when the stretch is not a "nice" number.

Activity

1. Read through Example 5 in Focus E on pages 36 & 37 in your text.
2. Complete Focus Questions 35 - 38 on pages 37 & 38.
3. Do CYU Questions 39 - 45 on pages 38 & 39.

When you have completed these questions, ask your on-site teacher to get the solutions for you from the Teacher's Resource Binder and check them against your answers. After you do this, if there is something you had trouble with and still do not understand, contact your on-line teacher for help.

After you do the assigned activities, click on the Test Yourself button at the top of the page for a quick quiz on this lesson.

Test Yourself

Find the transformational form of the parabola with vertex (-2, -3) and passing through the point (4, -8).

Solution