
Learning Resources
Outcomes
By the end of this lesson you will be able to answer questions like the ones in the pre-test below.
Approach #1
Answer the questions on the pre-test below in your Math 3103 binder. Then refer to the pages of the lesson that contain instruction on the questions you were unable to do, for which you got an incorrect answer, or for which you would like some further explanation.
Approach #2
Proceed directly to the first page of the lesson without attempting the pre-test. To do this simply click on the Lesson button above. You can then return to this pre-test later and use it as a worksheet.
Pre-test
Write each of the following numbers as a pure imaginary number in the form bi and simplify.
1. ![]() 2.
2. ![]() 3.
3. ![]() 4.
4. ![]()
For each of the following, perform the indicated operations and leave all i number answers in simplest terms:
5. ![]() 6.
6. ![]() 7.
7. ![]() 8.
8. 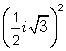
9. ![]() 10.
10. ![]() 11.
11. ![]() 12.
12. ![]()
13. Evaluate the following polynomial for the values of the variable given:
x3 - x2 + 4x - 4 for 2i and -2i
14. What do your answers in question 13 tell you about the function f(x) = x3 - x2 + 4x - 4 ?
Students will be expected to:
- Identify, add, subtract, and multiply complex numbers.
- Verify complex numbers as solutions to polynomial equations.
Introduction
This section consists of two lessons as follows:
Lesson 01 Pure Imaginary Numbers
This lesson introduces the concept of pure imaginary numbers and the use of the symbol i to represent them. It also shows how to add, subtract, multiply, and simplify pure imaginary numbers. It shows how to substitute pure imaginary numbers into polynomial equations and functions to verify that they are roots and zeros of each respectively.
Lesson 02 Complex Numbers
This lesson introduces the concept of complex numbers and the use of the symbol a +bi to represent them. It also shows how to add, subtract, multiply, and simplify complex numbers. It shows how to substitute complex numbers into polynomial equations and functions to verify that they are roots and zeros of each respectively.
Prerequisites
To be successful in this lesson, it would be helpful to know the following:
- Definition of and how to work with Real Numbers.
- Definition of and how to work with radicals which are real numbers.
- The meaning of the zeros of a function and the roots of an equation.